FLOW ASSURANCE: Part III: Coupled velocity, temperature fields in bundled pipelines

Coupled velocity and temperature fields.

In subsea applications where pipelines reside in extreme cold, waxes will precipitate out of solution to form beds, or perhaps, adhere azimuthally to the pipe wall, and hydrate crystals may aggregate to form immovable plugs. The erosive force imparted by the shearing action of the moving fluid may not be strong enough to assure smooth unimpeded flow. When this is so, expensive chemical remediation and external heating may be required.

In this series, we focus on the interaction between fluid-dynamical and thermal fields only. In the simplest physical limit when free convection effects are negligible, compared to forced convection, and flow occurs in the axial direction only, the equations of motion take on a tractable form amenable to mathematical solution. The properties of such flows can be explained rather straightforwardly.

Pipelines are often warmed by carrying within them separate lines that increase temperature by internal heating. "Pipes within pipelines" are known as "bundled pipelines." In the simplest case, a circular pipe might contain another pipe, which houses hot, flowing fluid isolated from the production flow. The inner pipe can, of course, be electrically heated too, or mounted to the side. The exact source of heat is not germane to our analysis.

Mathematical formulation

Velocity redistribution due to bed buildup.

The engineering problem yields simplifications. In the leading approximation, the temperature field T(x,y) satisfies Laplace's equation, with high temperatures prescribed at the inner pipe and cold ocean temperatures assumed at the outer walls.

The amount of heating required would depend on the thermodynamic properties of wax and hydrate appearance. The solution leads to a thermal field that varies rapidly in the flow domain, now an annular and not a pipe cross-section, that strongly affects momentum transport properties like viscosity.

More precisely, we must speak of "n," "k," and "yield stress," if general Herschel-Bulkley models are used to characterize wax or hydrate slurries.

Fortunately, the temperature equation and the modified flow equation for annular velocity can be solved using the finite difference scheme developed for "simple annuli" described in Part I. Only minor changes are required, since both differential equations are similar. Color graphics tools can also be modified to display both temperature and velocity. Finally, we ask, "How do typical solutions behave?"

Velocity, thermal fields

Azimuthal wax buildup around the pipe.

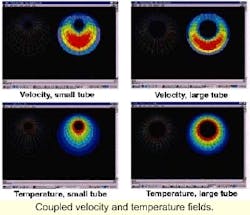
Left figures show computed solutions for velocity (top) and temperature (bottom) assuming a small inner heat pipe. "Red" denotes "fast" for velocities and "hot" for temperature. In contrast, "blue" represents "slow" and "cold." The right figures display calculated results for a hypothetical "large" inner pipe.

Consider the left figures. The velocity solution shows rapid flow speeds in the wide part of the annulus, as expected. For the temperature field, hot fluid surrounds the pipe, which rapidly adjusts to cold ambient conditions. In both cases, the use of advanced "boundary conforming, curvilinear" grids provide physical resolution in tight spaces as needed and especially in the rapidly changing regions adjacent to the inner pipelines.

Of course, which flow is "better" in practice is not apparent from color plots or grid displays. In order to determine economic viability, the volume throughput associated with the annular flow obviously enters. One might speculate that the higher the eccentricity, the better the production, since high eccentricities typically yield higher flow rates in many problems. But now this may not be true, since our transport coefficients are variable.

Secondly, production economics is determined by heating costs. In the problem formulated above, temperatures are fixed at inner and outer boundaries. In order to maintain the assumed steady state, a nonzero heat flux is required along the entire length of the pipeline. This can add significantly to operating costs. But this may be inexpensive, by comparison, to a "no heating" scenario where the pipeline clogs, even just once.

Any credible economic model must address the factors introduced above, in addition to costs associated with construction and down-time. Just as important, any useful mathematical model must be easy to use, allowing convenient input properties definition for the wax and hydrate slurries being modeled. This is crucial because engineers must consider numerous operating scenarios before optimal ones are identified.

Consider, for example, the flow exponent "n." The user enters a baseline value, say "1" for Newtonian flows or Bingham plastics. Also, the gradient "dn/dT" is also supplied, where "T" again denotes temperature. Such properties are obtained from laboratory measurement. Once the geometry of the pipe bundle is specified, the "Simulate" command is "clicked" and solutions appear within seconds.

"Real-world" effects

In order to keep the discussion simple, we ignored the presence of time-varying debris bed heights and growing plug-like obstacles. Of course, the transient buildup and erosion development process must not be neglected. Alterations in flow cross-section can affect the flow in unanticipated, serious, and subtle ways.

The picture at the left illustrates a "typical" annular flow, that is, with expected high "red" velocities in the wide part. The one at the right describes one possible flow pattern that arises with debris bed buildup.

The "red" zone displaces symmetrically in the lateral directions because the bottom annulus is dominated by the "no-slip" condition. The initial red zone now bifurcates into two small domains, differently from what is expected of "single pipe alone" flow. Because geometric similarity is completely lost, the new flow topology possesses completely different "flow rate versus pressure gradient" properties from the former.

In the top figure, we were concerned with beds that build preferentially at the bottom. However, "beds" may develop from above too, for example, the result of buoyant, low-density wax in high density fluids. We did not show deposition around the pipe, since the flow and ocean environment were assumed to be in thermal equilibrium.

Near the production well, equilibrium conditions may not apply. The flow may not be isothermal. Consequently, wax deposits about the entire inner surface, with a deposition rate that is proportional to the thermal gradient defined by pipe and ocean temperatures and the instantaneous layer thickness. Initially, debris buildup is quick. However, as the layer builds, the influence of the cold ocean is lessened and the growth rate decreases.

Another complicated model? Not quite. An interesting physical analogy can be drawn with mudcake buildup under static filtration in a Darcy flow. In the wax buildup illustration above, a "gray" mudcake is shown at the circular borehole face located in a cross-hatched Darcy formation, together with a "typically yellow" wax coating lining a circular pipe.

Here, the filter-cake growth rate is proportional to the rate at which solid particles are left behind, that is, to the local pressure gradient. As the cake thickens, this gradient lessens and the rate of growth slows. The mathematical analogy between wax growth in hot pipelines and mudcake growth in boreholes, it is seen, is almost exact, and models developed for the latter can be used to simulate a new subsea phenomenon. There are numerous models related to "formation invasion."

We have shown how the general annular equation solver and color display algorithms can be modified to solve for the coupled velocity and thermal fields typically encountered in bundled pipeline analysis. Importantly, good production economics depends on just the right combination of high flow rate and low heat flux usage. Detailed numerical simulations are required, but because fast mapping and convergence acceleration methods are now available, typical simulations - from input definition to final color output - can be completed within minutes.

Modern flow assurance analysis means more than studying rheological effects of wax and hydrate slurries in circular pipes. This is especially true because flow cross-sections are hardly circular. Wax deposition can form deep beds and hydrate aggregates can create immovable plugs. It has been shown that these "difficult" flows can be simulated conveniently. And, in general, computational methods are a "must," because the competing effects of nonlinear rheology, geometric complexity, and unsteady fluids and solids interaction, cannot be anticipated beforehand.

Author

Wilson C. Chin earned his PhD at MIT and his MSc from Cal Tech. He is affiliated with StrataMagnetic Software, LLC (Houston) and focuses on computational rheology, reservoir flow analysis, electromagnetic modeling, and software development. He may be contacted by email at "[email protected]."